
|
This movie shows the hypercube sliced square first, but here the faces of the slice and the cubes of the hypercube are colored so that you can tell which part of the slice comes from which part of the hypercube. The various faces of the slice sweep out the cubes of the hypercube (just as the edges of the slices of the 3D cube trace out the square faces of the cube). The first part of this movie shows two of the cubes of the hypercube (green and purple) being sweept out by the sides of the slice. Half-way through the hypercube, the slice leaves these two cubes and enters another pair of cubes (cyan and yellow) and the sides of the slice trace these out. We repeat the slicing sequence again, but this time highlight two other cubes (red and blue). These cubes share only an edge with the initial square slice of the hypercube, and as the slice moves through the hypercube, it cuts these cubes according to the edge-first sequence for the cube. The remaining two cubes (not shown) follow a similar pattern. | |
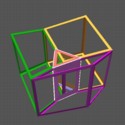
|
Here we see the hypercube cut edge first. Since there are three cubes of the hypercube meeting at an edge, these three cubes (green, purple, orange) begin to be sliced edge first. The slices are rectangles that grow in size, and these form the sides of a triangular prism. The slice progress through these cubes until they have been sliced half way through (one third of the way through the hypercube). At this point, the slice has reached thre more edges of the hypercube; it is passing through two of the cubes at each of these edges already, and so enters the third. This brings three more cubes into the picture (brown, cyan, yellow), each being cut edge first. For the next third of the sequence for the hypercube the slice passes through the rest of the original three cubes and gets half-way through the new three cubes. The six rectangles formed by slicing these cubes form the sides of rectangular prisms; half way through, the prism is a regular rectangular prism. When the prism again becomes a triangular one, the lower three cubes have been completed, and the slice continues on through the other three, finishing at their common edge -- the opposite edge in the hypercube from the one where the slice started. So far, we have tracked theslice through six of the eight cubes of the hypercube. The rest of the movie shows the slice passing through the other two. These are the ones that meet the original edge at a vertex; the red cube one top of the edge, and the blue one at the bottom. The slice of the hypercube enters these cubes corner first, and they go through the slicing sequence that starts at a triangle which grows, becomes truncated, then a regular hexagon, and reverses the process to shrink back to the opposite corner. These slices form the top and bottom faces of the prisms that make up the complete slice of the hypercube. | |

|
Finally, we slice the hypercube corner to corner. Four of the cubes meet the hypercube at a corner (blue, brown, cyan, yellow), so the initial slice cuts each of these cubes corner first. Each of these slices is a triangle, so the slice of the hypercube is a tetrahedron. This tetrahedron grows until it meets four corners of the hypercube, one third of the way through each cube, and one quarter of the way thorugh the hypercube. At each of these corners, the slice already is passing through three of the cubes at that corner, so it enters the fourth. This means the slice is now passing through all eight of the cubical faces of the hypercube. The slice enters these new cubes corner first, and starts as a small triangle. Meanwhile, the slices in the original four cubes become truncated triangles, and as the slice reaches half way through these cubes (one third of the way through the hypercube), these slices are regular hexagons. So four of the cubes are sliced as hexagons and four as triangles, forming an Archimedean solid, the truncated tetrahedron. As the slice progresses, the triangles grow and the hexagons move toward a triangle again, and half-way through the hypercube all eight cubes are sliced in exactly the same way; four are sliced 2/3 of the way through and the others 1/3 of the way through, but each is sliced as an equailateral triangle. These eight triangles form a regular Platonic solid: the octahedron. From here, the sequence of shapes is seen again but in reverse. The triangles in the original four cubes shrink down to a point (at the corner of the cube opposite to the one where the slicing started), and the slice through the others pass first through truncated triangles, then the hexagon, then truncated triangles and back to a triangle again. The slicing of the original four cubes is now complete, while the other four are now 2/3 complete (and the hypercube itself is 3/4 complete). We end as we began, with tetrahedra shrinking to a corner, the one in the hypercube that is opposite to the one where the sequence began, though both appear at the center in the projection. |