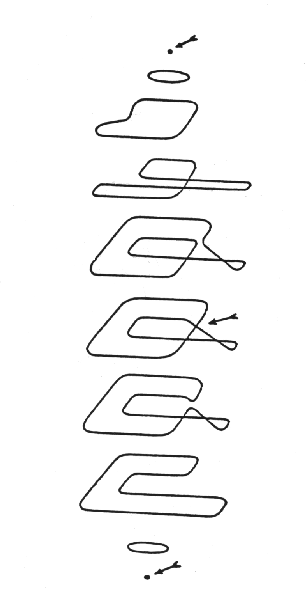

Kuiper described an immersion of the projective plane by giving the levels sets for different heights of a polar height function, as in the following picture:


Here, the arrows point to the three critical points. The difficult transition to visualize is the one between the third and fourth levels from the top. This is explained in more detail at the right, where the red segment is moved across the upper loop to the purple position. The triple point is formed as the red line moves through the crossing formed by the upper loop. The complete surface can be obtained by "filling in" bands of surface between the different levels.
A smooth tight surface has the property that all its positive
curvature must be on its
convex envelope,
so everything inside the convex envelope must have negative or zero
curvature
![[More]](pix/Link.gif) .
Kuiper's idea was to try to build the projective plane so
that, if he cut off the maximum at the top and the minimum at the
bottom, the rest would be negatively curved; this would form the
central core of a tight immersion of a projective plane with a handle
(it would be pasted into a big sphere with two disks removed, which
would form a handle from the top to the bottom, and would make the
surface tight).
.
Kuiper's idea was to try to build the projective plane so
that, if he cut off the maximum at the top and the minimum at the
bottom, the rest would be negatively curved; this would form the
central core of a tight immersion of a projective plane with a handle
(it would be pasted into a big sphere with two disks removed, which
would form a handle from the top to the bottom, and would make the
surface tight).
Kuiper tried to accomplish this by using pieces of ruled surfaces to connect the level sets that he had drawn, and since every point on a ruled surface has a direction with zero curvature, the curvature is everywhere non-positive. The only possible problem would occur at the interfaces between two pieces of ruled surface where they join along one of the level sets.

Unfortunately, Kuiper could not connect the ruled surfaces without introducing some positive curvature between the points A and B in the diagram. To overcome this problem, he introduced an additional handle (inset at right) that attached to the outer shell, thus removing the area of positive curvature from the central core.
This model serves as the basis for the tight immersions of the remaining non-orientable surfaces: handles can be added to this surface as before in order to obtain tight immersions of any surface with odd Euler characteristic less than -3.
![[Right]](pix/Right.gif) There is no tight projective plane
There is no tight projective plane
![[Left]](pix/Left.gif) Non-orientable tight surfaces
Non-orientable tight surfaces
![[Up]](pix/Up.gif) Kuiper's initial question
Kuiper's initial question
![[Up]](pix/Up.gif) The level sets of the polyhedral solution
The level sets of the polyhedral solution
7/20/94 dpvc@geom.umn.edu --
The Geometry Center