by Nobody N. Particular
Life is filled with uncertainty. Nobody knows if it's going to rain tomorrow, if a comet might land on Washington, if I'll meet the person I'm going to merry, or what will happen if I toss a coin.
Well, OK, we do know some things about what will happen when I toss the coin. Gravity will make it hit the ground. Or some horizontal surface like a tabletop. It won't land on it's edge. It will land on heads so tails will be up. Or it will land on tails so heads will be up.
There's no rime or reason for heads instead of tails. So I started thinking if the coin tossing might be chaos. I tried it, and this is what I got.
How to tell if something is chaos? I looked in chapter 6 of the book. I decided to try the Kelly pictures. This was the test I made the most sense of.
I need many numbers to tell chaos. They said this in class. This puzzled me at first. I asked the teacher and was told if you just have two numbers, how can you tell if it's chaos or not? Two numbers aren't enough to tell anything. How many different pieces of music start with the same first two notes? Lots, I bet, unless the notes are pretty wierd. What's that old game show - Name That Tune? So I figured I'd toss the coin a lot of times - I did 400. I'd toss the coin, see how it landed, and write it down. At first, I wrote down "heads" or "tails." But that was to much writing. So I just wrote down "H" or "T". I wasn't sure if writing the whole word or just the initial would make any difference. So I started the experiment over when I used the initial.
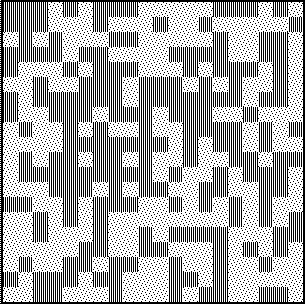
And now for the lucky part of my work. A Kelly picture has to be put in a square. Because otherwise it doesn't look right. So how can I find a square to put 400 coin tosses in? You make a little square for each toss. $00 tosses, so 400 little squares. You put the squares down just like you read english. Start at the left side of the top line and put squares down, one after another, till you get to the end of the top line. The next square goes on the left side of the second line. Keep putting down squares (you know, not insulting them, but just putting them in their place) till you get to the end of the second line. The next square goes on the left side of the third line. Keep putting down squares till you fill the third line. Do you get the picture now? This wasn't so clear in the book. I tried hundreds and hundreds of things till I found this. But I'm glad, because I finally figured it out. Now there are two more problems to figure out. And it's really the next problem where I was so lucky. How can I put all 400 of my little squares into a big square? To save time, I decided to only look at lines that had 5, 10, 15, 20, 25, 30, 35, or 40 little squares in it. If there are 5 little squares in a line, I found I needed 80 lines. This makes the Kelly picture not a square at all, but a long, thin rectangle. !0 little squares in a line is better. I only need 40 lines. So it looks like the more little squares in a line, the closer the Kelly picture is going to be to a square. Encouraged by this hypothesis, I tried 15 squares in a line. You'll never beleive what I found. I filled up 26 lines and had 10 squares left over!!! Now I know how Ptomaine felt when Galileo proved him wrong that the earth does go around the sun. All this hard work. I cut out all these little squares and put them on my desk. And the problem was harder than even that. My roommate's stupid cat kept jumping on the desk, scattering the squares to the fore winds all over creation. If we lived on the second floor, I'd throw the cat out the window. But we only live on the first floor, so it wouldn't make any difference. Finally, I locked the cat in my roommate's closet and finished my project. Where was I? O yes. A line with 15 squares doesn't work. Filled with despair, I was about to quit and do another project (Except of course it's the night before the project is due, and I have an exam in another class tomorrow too, and I've got to start studying sometime.) Then I tried a line with 20 little squares. Amazing! It filled out a whole square, AND THE SQUARE HAS 20 LINES! Do you see what that means? This will make a square Kelly picture. My worries are over. How lucky it was that I tried 400 tosses. If I'd done 399, making a square might have been a lot harder.
Finally how to color the H squares and the T squares. First I tried black and white, but this looked too much like a scrambled up checkerboard. Then I tried vertical stripes and horizontal stripes. But this looked too busy. Then I hit on the idea of trying vertical stripes and dots. Now I no that high fashion says you never mix stripes and poka dots. But this isn't fashion. It's science. How many scientists dress well? Well, OK the guy in Jurassic Park looked pretty cool. But most scientists dress like geeks. Just look around you and you'll see that I'm right.
But this is a little bit beside the point. Now I know I'm going to
toss the coin 400 times and do a Kelly picture 20 squares in a line 20
lines stripes for heads dots for tails. Here's what I got.

How do you understand this picture? Long horizontal rows of stripes means heads came up a lot in a row. For example, the first three tosses gave heads. Then a tail, then a head, then another tail, then three heads, then five tails, then three heads, and so on.
What do we see? It doesn't repeat head, tail, head, tail, head, tail, for ever and ever. It doesn't repeat head, head, tail, head, head, tail, head head, tail, for ever and ever. I tried lots of other patterns, and none of them got repeated. Try it yourself, if you don't believe me. So there's no pattern, so it must be completely random, so it must be chaos. I looked up "chaos" in the Random House dictionary and found "without pattern or order." And who would know more about randomness than the Random House dictionary. This made more sense than the definition in the book, so this is the one I'm going to use.
So my conclusion - coin tosses are a good, simple way to generate chaos that anybody can understand. What's the deal with all these dripping faucets and bouncing balls and populations of insects. And who cares about that stuff anyway? Like I care if two bugs kill themselves fighting over a blade of grass. But money - everybody cares about money. So I say, hay buddy, can you spare some chaos?
References
D. Peak, M. Frame, Chaos Under Control
Random House Dictionary
Go to Project 2
Return to Projects
Return to Chaos Under Control